
I had the opportunity to calculate the flow from the piping under the tank, so I will leave a note about the calculation method.
This time, we are calculating the outflow flow rate, outflow velocity from the piping, and pressure loss.
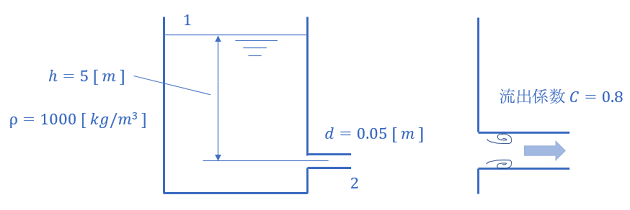
Height of tank bottom piping and tank water surface : 5 m
Tank bottom piping diameter : 0.05 m
Fluid density : 1000 kg/m3
Runoff coefficient : 0.8
*If you use this article as a reference for calculations, please do so at your own risk. We cannot be held responsible for any troubles caused by this article.
Calculation method for tank outflow flow rate, flow velocity, and pressure loss (Bernoulli’s theorem)
What is the flow velocity at the pipe outlet?
Assuming that there is no loss between the tank water surface and the pipe outlet, Bernoulli’s theorem holds true.
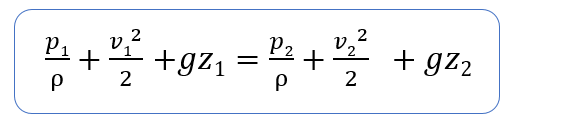
p1 and p2 are atmospheric pressure, p1=p2.
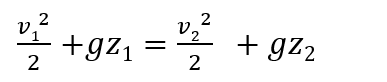
Since z1=h, z2=0,

Also, since there is no velocity at the tank water surface, v 1 =0.
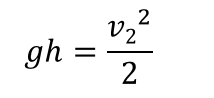
Find the flow velocity at the pipe outlet from the above equation.
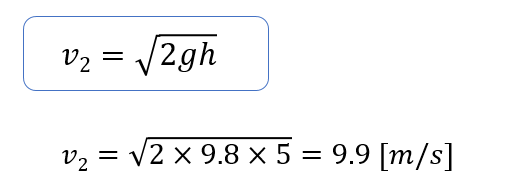
We were able to obtain v2=9.9 [m/s].
What is the outflow flow rate at the pipe outlet?
From the continuity equation, the flow rate is below.
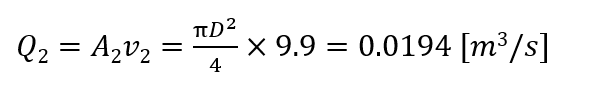
The actual outflow flow rate is the result obtained above multiplied by the outflow coefficient C,
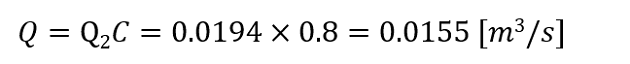
The outflow flow rate was determined to be 0.0155 [m3/s].
How many energy losses?
When there is energy loss, the following energy conservation law holds between cross sections 1 and 2.

If we rearrange the formula from the given conditions,
(p1=p2, z1=h, z2=0, v1=0)
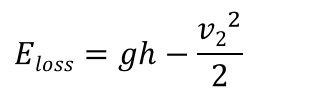
The flow velocity at the tank piping outlet is given by the outflow flow rate Q and the cross-sectional area A2.
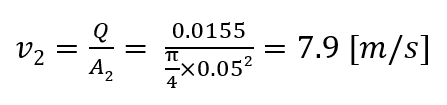
Substituting v2 obtained here into the energy loss formula,
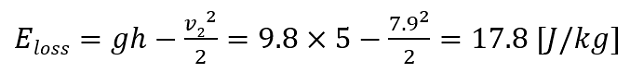
We were able to find the energy loss as shown above.
.
Appendix: Unit conversion (energy loss → pressure loss)
Energy loss is represented by pressure loss.

The calculation units are shown below for your reference.

summary
I think you can calculate the flow rate, flow velocity, and pressure loss from the tank bottom piping using the calculation formulas introduced so far.
This time, the contraction coefficient and runoff coefficient are given as initial conditions, but in reality, there may be data obtained from experimental values or reference values unique to universities or companies.
I didn’t have reliable data or numbers on hand, so I referred to commonly found numbers. (This is because it focuses on how to do calculations.)
The above is a calculation memo about how to calculate the fluid flowing out from the tank bottom piping. I would appreciate it if you could refer to it.



コメント